Frodo - a 10 inch travel Dobson

10 inch, f/4.8 newtonian

Telescope Making
Web Ring

home

features!

numbers

basic considerations

optics
hogging out
smoothing the curve
polishing
foucault tester
figuring
silvering

mechanics
mirror cell
wire spider
focuser
middle ring
rocker box

travel and assembly

performance

acknowledgements

basic considerations

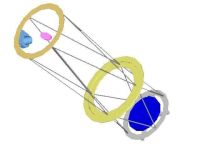
Building a travel Dobson usually means going for lightness.

I’ve thought about my habits and preferences and realized, I much more like to be able to watch the skies comfortably (couch potato that I am) than to take my telescope on a bicycle through the alps, set it up in 2 minutes and lie down for altitudes less than 20°.

I want to use 2 inch eyepieces and have all the comfort of a homebound telescope.

But still I wanted the telescope portable because when I travel with my family I thought it would be nice to have a telescope I can take along, set up once at our destination and take apart at the end of the holiday.

It must be air travel compatible.

For comfort of viewing the traditional Dobson design with a fairly high centre of gravity resulting in a tall rockerbox really appealed to me. It means when watching the skies the eyepiece moves on a hemisphere with an elevation equal to the height of the rotational axis and a diameter equal to the distance of the eyepiece from that axis.

The closer that COG is to the eyepiece, the smaller the hemisphere and the less I would have to move to jump from object to object.

Even more comfortable is to be able to rotate the tube assembly along its long axis for easiest viewing position. This is usually realized with a tube in a rotation mounting cradle.

I didn’t want a solid tube because that would be way too bulky to take along so it had to be a truss tube design.

On the internet I came across the very nice homepage of Steven Lee and his explanation on why most Serrurier truss telescopes really are not what they call themselves. It is the back to back arrangement of the triangular trusses connecting the upper tube assembly including secondary & focuser and the lower tube assembly including mirror and cell with a central element that is connected to the support, that makes a genuine serrurier truss. The ingenious principle makes sure the optics stay aligned even though the secondary and the main mirror sag when going from high to low altitudes by making sure they sag the same amount.

The idea of a three ring construction formed and I thought it would solve a lot of the construction problems and provide the functionality I wanted.

This is the design I came up with:

The single upper ring with a wire spider and a self-made low profile 2 inch Crayford focuser is connected to a flexible birch plywood ring (9mm), which in turn is supported by a stronger plywood ring (19mm) which provides the necessary rigidness and attaches to the altitude bearings (not shown). The flexible ring can rotate inside this ring. The 6 point mirror cell (calculated with PLOP) is made from aluminium square tubing (20mm) and for easier collimation provides four connections to the lower plywood ring. This design is strongly influenced by Steven Lees wonderful 12 inch scope.

The collimation thumbscrews face upwards and are easily accessibly. Since the focuser and the collimations screws align, it is very easy to collimate. One knows exactly which screw has to be turned to tune the alignment. This principle also applies to the wire spider.

If my thinking was right the overall design should provide the following advantages:

I did a few FEM calculations using the evaluation version of a program called Prostab, written by the German engineer Ernst Sauer. I very much appreciate the free use of the evaluation version and especially his help in setting up the input file as Prostab is usually used by civil engineers (they use weird units, well, at least weird to me...).

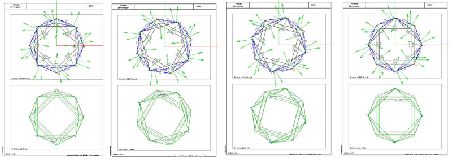To match the sag of the upper and lower ring I found it best to use 15 mm diameter aluminium trusses (2 mm wall thickness) for the UTA and 10 mm diameter (1 mm wall thickness) for the LTA. This calculation is based on the assumption of ideal truss connections and yields sag of not more than 0.03 mm at both ends (with the telescope pointing at 0° altitude and different rotation angles around the lateral telescope axis). These results made me realize the sag caused by the static loads on the truss construction is so small, that it is unnecessary sophistication to choose different tube diameters and wall thickness. I bought readily available standard aluminium tubing from the DIY store with 15 mm diameter and 1 mm wall thickness. Recalculation with these values gave me maximum sag of 0.05 mm at the upper end and 0.01 mm at the lower end, so taking the telescope from 90 deg alt to 0 deg will result in a parallel misalignment of 0.04 mm, which I hope to find acceptable. Other effects like the imperfect real world truss connections that I can realize with my handheld DIY tools will dominate the amount of sag. Still I hope that by using this kind of construction the unavoidable imprecision in construction will cancel each other out.